Question

Use the ratio form of Kepler’s third law,
`( (Ta)/(Tb) )^2` =
`( (Da)/(Db) )^2`, and the data provided to determine the time it takes Mars to orbit the Sun. Round your answer to the nearest tenth.

Earth’s orbital period = 1.0
Earth year Earth’s distance from the Sun = 1.0 AU
Mars’s distance from the Sun = 1.5 AU

Answer 1

the awnser is that it takes 1.8 earth years for mars to orbit the sun

Answer 2

Answer: Time period taken by Mars to orbit round Sun is 1.8 earth years

Step-by-step explanation:

Earth’s orbital period =
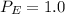`P_E= 1.0` Earth year

Earth’s distance from the Sun =
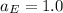`a_E =1.0`AU

Mars’s orbital period = ?
`=P_M`

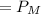
Mars’s distance from the Sun =
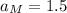`a_M= 1.5`AU

According to Kepler third law:

`P^2\propto a^3`

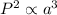
P = time period taken by the planet to orbit Sun

a = semi major axis between the planet's orbit

So, we can rewrite it as:

`((P_E)^2)/((a_E)^3)=((P_M)^2)/((a_M)^3)`

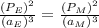
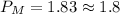`P_M=1.83\approx 1.8` earth years

Time period taken by Mars to orbit round Sun is 1.8 earth years