Question

Write each equation in slope-intercept form6x+9y=277x=3y-12

Answer 1

The slope-intercept equation of a line is given by

y = mx + b

where m is the gradient, and b the intercept

So w can re-write the two equations in this form by making y the subject of the formula.

Given: 6x + 9y = 27

Step 1: make 9y the subject of the formula

9y = -6x + 27

Step 2: Divide both sides by 9

`\begin{gathered} y\text{ =}(-6x)/(9)\text{ + }(27)/(9) \\ \\ y\text{ = -}(2)/(3)x\text{ + 3} \end{gathered}`

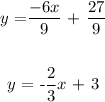
Slope Equation of the first equation => y = -2x/3 + 3

For the second equation

Given: 7x = 3y - 12

Step 1: make 3y the subject of the formula

3y = 7x + 12

step 2: Divide both sides by 3

`\begin{gathered} y\text{ =}(7x)/(3)\text{ + }(12)/(3) \\ \\ y\text{ =}(7x)/(3)\text{ + 4} \end{gathered}`

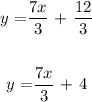
Slope Equation of the first equation => y = -2x/3 + 3

The slope equation of the second equation is => y = 7x/3 + 4