Question

PLEASE HELP.find the solutions for a triangle with a =16 c =12 and b=63 degrees

Answer 1

a.16
b.12
c.63
try to have the triangle and draw a little drawling it will help
and start from there

Answer 2

Side b is 15.02,

Angle C is 45.39° and angle A is 71.61°

Explanation:

Let in triangle ABC,

BC = a = 16 unit,

AB = c = 12 unit,

∠B = 63°,

By the law of cosine,

`b^2=a^2+c^2-2ab cos B`

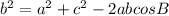
By substituting the values,

`b^2 = 16^2+12^2-2* 16* 12 * cos 63^(\circ)=256 + 144 - 384 cos 63^(\circ)=225.67`

`\implies b\approx 15.02`

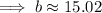
By the law of sine,

`(sin B)/(AC)=(sin C)/(AB)`

`(sin 63^(\circ))/(15.02)=(sin C)/(12)`

`\implies sin C= (12sin 63^(\circ))/(15.02)= 0.7119`

`\implies \angle C\approx 45.39^(\circ)`

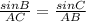
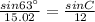
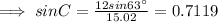
∵ The sum of all interior angles of a triangle is supplementary,

⇒ ∠A + ∠B + ∠C = 180°

⇒ ∠A + 63° + 45.39° = 180°

⇒ ∠ A + 108.39° = 180°

⇒ ∠A = 71.61°