Question

Use the Law of Sines to solve (if possible) the triangle. If two solutions exist, find both. Round your answers to two decimal places. (If not possible, enter IMPOSSIBLE.)A = 111°, a = 25, b = 24

Answer 1

`a=25,m\angle A=111^(\circ),b=24,\angle B=63.67,c=2.49,\angle C=5.33^(\circ)`

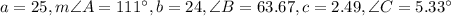
1) Let's begin by sketching out this triangle to beter gras p it:

2) So now, we can write out the Law of Sines formula:

`\begin{gathered} (a)/(\sin(A))=(b)/(\sin(B))=(c)/(\sin(C)) \\ \\ (a)/(\sin(A))=(b)/(\sin(B)) \\ \\ (25)/(\sin(111))=(24)/(\sin(B)) \\ 25\sin(B)=24\sin(111) \\ (25\sin \left(B\right))/(25)=(\sin \left(111^(\circ \:)\right)\cdot \:24)/(25) \\ \sin \left(B\right)=(\sin \left(111^(\circ \:)\right)\cdot \:24)/(25) \\ B=\arcsin \left((\sin \left(111^(\circ \:)\right)\cdot \:24)/(25)\right) \\ m\angle B=63.67 \end{gathered}`

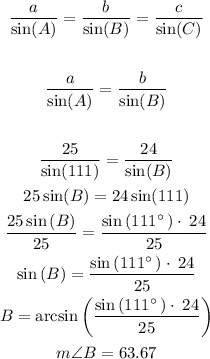
Note that we picked two ratios to find one variable. So now, let's find the measure of the angle C:

Since we know angle A, and angle B let's find the angle C by using the Triangle Sum Theorem:

`\begin{gathered} m\angle A+m\angle B+m\angle C=180 \\ 111+63.67+m\angle C=180 \\ 174.67+m\angle C=180 \\ m\angle C=180-174.67 \\ m\angle C=5.33 \end{gathered}`

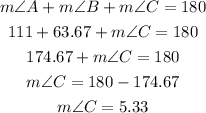
3) Now, let's find the one missing the leg c:

`\begin{gathered} (a)/(\sin(A))=(c)/(\sin(C)) \\ (25)/(\sin(111))=(c)/(\sin(5.33)) \\ (c)/(\sin \left(5.33\right))=(25)/(\sin \left(111^(\circ \:)\right)) \\ (c\sin \left(5.33\right))/(\sin \left(5.33\right))=(25\sin \left(5.33\right))/(\sin \left(111^(\circ \:)\right)) \\ c=(25\sin \left(5.33\right))/(\sin \left(111^(\circ \:)\right)) \\ c=2.49 \end{gathered}`

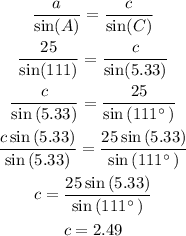
Note that we rounded off to the nearest hundredth.