Question

Hello, I need help with this precalculus homework question, please?HW Q3

Answer 1

Given

`R(x)=(13x+13)/(8x+16)`

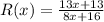
To find:

a) The domain of R(x).

b) The vertical asymptote.

c) The horizontal asymptote.

Step-by-step explanation:

It is given that,

`R(x)=(13x+13)/(8x+16)`

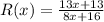
a) Consider

`\begin{gathered} 8x+16\\e0 \\ \Rightarrow8x\\e-16 \\ \Rightarrow x\\e-(16)/(8) \\ \Rightarrow x\\e-2 \end{gathered}`

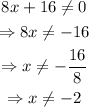
Hence, the domain of R(x) is,

`\lbrace x|x\\e-2\rbrace`

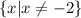
b) To find, the vertical asymptote set the denominator equal to 0 and solve for x.

`\begin{gathered} \Rightarrow8x+16=0 \\ \Rightarrow8x=-16 \\ \Rightarrow x=-(16)/(8) \\ \Rightarrow x=-2 \end{gathered}`

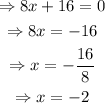
Hence, the vertical asymptote is x=-2.

c) To find the horizontal asymptote set y as the fraction of the coefficients of x in the numerator and the denominator.

`\Rightarrow y=(13)/(8)`

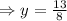
Hence, the horizontal asymptote is 13/8.

Thus,

a) The domain is,

`\lbrace x|x\\e-2\rbrace`

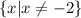
b) The vertical asymptote is x=-2.

c) The horizontal asymptote is y=13/8.