Question

A certain spinner has equally sized slices. The adds in favor of landing on a green slice are 7:2. What is the probability of landing on A green slice

Answer 1

Given: The odds in favor of landing on a green slice are 7:2

To Determine: The probability of landing on A green slice

Find the total number of landing slices

Since the ratio given is 7:2. The total number of landing slices would be a multiple of the total ratio. The total ratio is

`\text{Total ratio=7+2=9}`

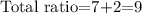
Therefore, the total number of equal slices is a multiple of 9

Find the ration of the odds on green slice

The ration of the odds on green slice given that the ratio of the odds in favour of landing on a green slide is 7:2 would be multiples of 7

Find the probability of landing on A green slice

The probability of an event A from a sample space S is

`\begin{gathered} P(A)=(n(A))/(n(S)) \\ P(A)=\text{Probability of event A} \\ n(A)=\text{ number of element in event A} \\ n(S)=\text{ number of elements in the sample space} \end{gathered}`

The probability of landing on a green slice would be

`\begin{gathered} P(G)=(n(G))/(n(T_r)) \\ P(G)=\text{Probability of landing on a gre}en\text{ slice} \\ n(G)=\text{ number of multiples of the ration for a gr}een\text{ slice landing} \\ n(T_r)=\text{ number of multiples of the total ratio of the landings} \\ \text{Therefore:} \\ n(G)=7 \\ n(T_r)=9 \\ P(G)=(7)/(9) \end{gathered}`

Hence, the probability of landing on a green slice is 7/9