Question

HELP!!! SEE ATTACHED

If f is a function such that the quotient of the quantity f of b minus f of a and the quantity b minus a equals 2, then which of the following statements must be true?

f(a) = f(b) = 2
The slope of the tangent line to the function at x = a is 2.
The average rate of change of the function on the interval [a, b] is 2
The linear approximation for f(x) at x = a is y = 2

Answer 1

The average rate of change for the function on the interval [a,b] is 2

Answer 2

Explanation:

The given expression is

`(f(b)-f(a))/(b-a)=2`

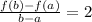
Notice that
`a` and
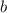`b` are elements of the domain and
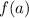`f(a)` and
`f(b)` are elements of the range.

Observe the notation used, an element of the range is always written like
`f(x)` where
`x` is an element of the domain.

Also, notice that this expression is using two pair of coordinates, which are
`(a, f(a))` and
`(b,f(b))`.

On the other hand, the definition of average rate of change is

`r=(y_(2) -y_(1) )/(x_(2)-x_(1) )`

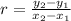
Where
`(x_(1) ,y_(1) )=(a ,f(a))` and
`(x_(2) ,y_(2) )=(b ,f(b))`.

Having said that, the given expression represents an average rate of change in the interval, where the rate is 2. The right answer is the third option.