Question

Complete the remaining steps of the proof:given: -angle QSR is congruent to angle TRS-side PR is congruent to side PSProve:triangle QSR is congruent to triangle TRS. StatementsReasons1. Given1. PR = PSZQSR *ZTRS2. ZTSR * ZQRS 2.I3. SR RS3.4. AQSR SATRS4.Corresponding parts of congruent triangles arecongruent:: Base angles of an isosceles triangle are congruentReflexive property:: Side-Angle-Side (SAS) postulateAngle-Side-Angle (ASA) postulate

Answer 1

Write out the given parameter for the proofing

Given:

`\begin{gathered} \Delta QSR\cong\Delta TRS \\ \vec{}PR\cong\vec{}PS \end{gathered}`

From the given parameters, it can be seen that triangle PSR is an isosceles triangle because two sides (line PR and line PS) are equal

An isosceles triangle has two equal base angles.

`\angle TSR\cong\angle\text{QRS(base angles of isosceles triangle are equal)}`

Triangle QSR and triangle TRS has a common side which is line SR and line RS. This property is reffered to as reflective property

`\vec{}SR\cong\vec{}RS(reflective\text{ property)}`

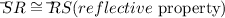
From the above proofs, it can be seen the triangle QSR and triangle TRS have a same side and same angles

Therefore,

`\Delta QSR\cong\Delta TRS(angle-side-angle(ASA)postulate)`

Nothing