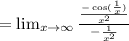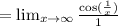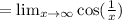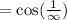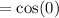Answer:
The exact value is 1.
Explanation:
Here, given expression,
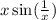
When we put, x = 5, 10, 15, 20, 25, 30, 35, 40, 45, 55,.....
In the expression,
We found that the value of the expression is approaches to 1,
Hence, the exact value of,
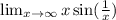 is 1.
is 1.
Alternative method :
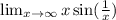
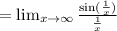
By L'hospital's rule,