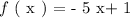Question

Find an equation of the line passing through the given points (-3,16) and (1,-4) . use function notation to write the equation .

Answer 1

Step 1:

In this question, we are meant to find the equation of the line passing through the given points:

`\begin{gathered} (x_1,y_1)\text{ = ( - 3, 16 )} \\ (x_2,y_2\text{ ) = ( 1, - 4)} \end{gathered}`

Step 2:

First, we need to calculate the gradient of the two points:

`m\text{ = }(y_2-y_1)/(x_2-x_1)`
`\begin{gathered} m\text{ = }\frac{-4\text{ - 16}}{1-(\text{ -3)}} \\ m\text{ =}(-20)/(4) \\ m\text{ = -5} \end{gathered}`

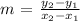
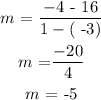
Step 3:

Using the formulae:

`\begin{gathered} y-y_{1\text{ }}=m(x-x_1) \\ \text{where x}_1\text{ = -3} \\ y_1=\text{ 16} \\ m\text{ = -5} \end{gathered}`

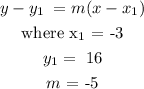
Putting the values into the equation, we have that:

`\begin{gathered} y\text{ - 16 = - 5 ( x - (- 3)} \\ y\text{ - 16 = -5 ( x + 3)} \\ y\text{ - 16 = -5x - 15} \\ \text{collecting like terms, we have that:} \end{gathered}`
`\begin{gathered} y\text{ + 5 x = - 15+ 16} \\ y\text{ + 5 x = 1} \end{gathered}`

CONCLUSION:

The function notation to write the equation is given as:

`y\text{ = -5x + 1}`

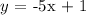
since y = f ( x ), then

`f\text{ ( x ) = - 5 x+ 1}`