Question

The graph of a line on a coordinate plane has a slope of 1/2 and passes through the point (4, -3). Which equation describes the line?

Answer 1

`\boxed {\boxed {\sf y= (1)/(2)x-5 }}`

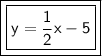
Explanation:

Since we are given a point and a slope, we should use the point-slope equation.

`y-y_1=m(x-x_1)`

where m is the slope and (x₁, y₁) is the point the line passes through.

We are given the slope of 1/2 and the point (4, -3). Therefore:

`m= (1)/(2) \\x_1= 4\\y_1= -3`

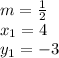
Substitute the values into the formula.

`y--3= (1)/(2) (x-4)`

`y+ 3= (1)/(2) (x-4)`

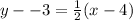
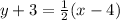
Distribute the 1/2. Multiply each term inside the parentheses by 1/2.

`y+3= (1)/(2) *x + (1)/(2) * -4`

`y+3=(1)/(2)x-2`

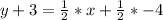
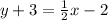
We want to the equation of the line in slope-intercept form or y=mx+b. We need to isolate y. 3 is being added and the inverse of addition is subtraction. Subtract 3 from both sides of the equation.

`y+3-3=(1)/(2)x-2-3`

`y=(1)/(2)x-2-3\\y= (1)/(2)x-5`

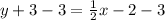
The equation of the line is y=1/2x-5