Question

Solve the equation for all values of x by completing the square

Answer 1

5x^2 - 20x = 60

5x^2 - 20x - 60 = 0

Using this fomulas:

`ax^2+bx\text{ + c = 0}`
`a(x+d)^2\text{ + e = 0}`
`d\text{ = }(b)/(2a)`
`e\text{ = c - }(b^2)/(4a)`

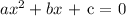
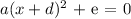
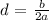
We can complete the square

a = 5

d = -20/(2)(5) = -20/10 = -2

e = -60 - (-20)^2/4(5) = -60 - 400/20 = -60 - 20 = -80

Completing the square:

5(x -2)^2 - 80 = 0

Solving for x:

5(x - 2)^2 = 80

(x -2)^2 = 80/5 = 16

(x - 2) = sqrt(16) = +4 and -4

for +4:

x - 2 = 4

x = 4 + 2 = 6

x = 6

for -4:

x - 2 = -4

x = -4 +2 = -2

x = -2

Answer:

The values of x are 6 and -2