Question

In the country of United States of Heightlandia, the height measurements of ten-year-old children are approximately normally distributed with a mean of 53.2 inches, and standard deviation of 3 inches.What is the probability that the height of a randomly chosen child is between 49.8 and 60.6 inches? Do not round until you get your your final answer, and then round to 3 decimal places.

Answer 1

Step-by-step explanation

Given that the mean is 53.2 inches, and standard deviation of 3 inches. We will first find the z scores for height 49.8 and 60.6. inches

`\begin{gathered} z=(x-\mu)/(\sigma) \\ z_(49.8)=(49.8-53.2)/(3)=-1.13333 \\ z_(60.6)=(60.6-53.2)/(3)=2.46667 \end{gathered}`

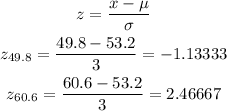
Therefore,

Using a z score calculator, The probability that the height of a randomly chosen child is between 49.8 and 60.6 inches is

[tex]P\left(49.8Answer: 0.865