Question

System of equations 3X-4Y=13 -6X+8Y=4 a. infinite #b.0c.1d.2e.3

Answer 1

GIVEN:

We are given the following system of equations;

`\begin{gathered} 3x-4y=13-----(1) \\ \\ -6x+8y=4-----(2) \end{gathered}`

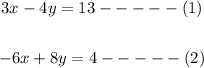
Required;

To determine whether or not the equations has a solution or no solution.

Step-by-step solution;

We can solve this system of equations by the elimination method. This is because none of the variables has 1 as its coefficient.

First step, we multiply equation (1) by -6 and then multiply equation (2) by 3 (that is, the coefficients of x in both equations).

`\begin{gathered} -18x+24y=-78-----(3) \\ \\ -18x+24y=12------(4) \end{gathered}`

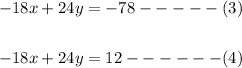
Next step, we subtract equation (4) from equatio (3);

`\begin{gathered} -18x-(-18x)+24y-24y=-78-12 \\ \\ -18x+18x+24y-24y=-90 \\ \\ 0+0=90 \\ \\ However; \\ \\ 0\\e90 \end{gathered}`

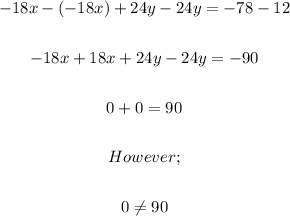
As we have seen from the calculations above, the result shows 0 equals 90 on both sides of the equality sign and that is NOT possible.

Therefore, for the given system of equations, there is NO SOLUTION

ANSWER:

Option B, that is 0 solutions is the correct answer.

Further Explanation;

For the graphs shown above, the color codes are as follows;

`\begin{gathered} Green:3x-4y=13 \\ \\ Red:-6x+8y=4 \end{gathered}`

This simply tells us that for both equations, there is no point at which they can intersect and therefore,