Question

I need help answering thisIf you can, simply show your work

Answer 1

we have that

To find the sum of the first Sn terms of a geometric sequence use the formula

`S_n=(a_1\cdot(1-r^n))/(1-r)`

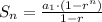
where

a1=120 -----> first term

n=8

Find out the common ratio r

we have

a1=120

a2=-80

a3=160/3

so

a3/a2=(160/3)/(-80)=-2/3

a2/a1=-80/120=-2/3

so

r=2/3

substitute given values in the formula

`S_8=(120\cdot(1-(-(2)/(3)^8)))/(1+(2)/(3))`
`S_8=\frac{120\cdot(1-(256)/(6,561)^{})}{(5)/(3)}`
`\begin{gathered} S_8=72\cdot((6,305)/(6,561)^{}) \\ S_8=(453,960)/(6,561)^{} \end{gathered}`

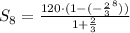
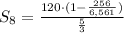
Simplify

S_8=50,440/729