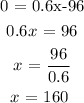Answer:
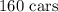
Step-by-step explanation:
Here, we want to get the number of cars to be made so as to minimize the unit cost
What we have to do here is to find the first derivative of the given cost function
Mathematically, we have that as:
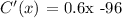
To get the minimum x value, we simply set the first derivative to zero and solve for x
Mathematically, that would be: