Question

Fill in the blanks below.Find the slope of the line passing through the points (-8, 4) and (-8. - 9).slope: ___

Answer 1

The slope of a line that passes through two given points A and B, is expressed as

`\begin{gathered} slope=(y_2-y_1)/(x_2-x_1) \\ where \\ (x_1,y_1)\text{ and \lparen x}_2,y_2)\text{ are the coordinates of the points through} \\ which\text{ the line passes.} \end{gathered}`

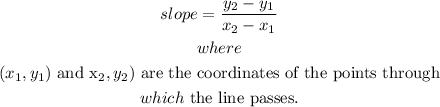
Given that the line passes through the points (-8, 4) and (-8, -9), this implies that

`\begin{gathered} x_1=-8 \\ y_1=4 \\ x_2=-8 \\ y_2=-9 \end{gathered}`

By substituting these values into the slope formula, we have

`\begin{gathered} slope\text{ = }(y_2-y_1)/(x_2-x_1) \\ =(-9-4)/(-8-(-8)) \\ =(-9-4)/(-8+8) \\ =(-13)/(0) \\ \Rightarrow slope=\infty \end{gathered}`

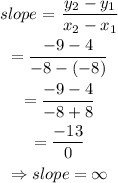
Hence, the slope of the line that passes through the points (-8, 4) and (-8. - 9) is evaluated to be

at infinity.