Question

The midpoint of MN is point P at (–4, 6). If point M is at (8, –2), what are the coordinates of point N?

Answer 1

The midpoint of a line segment is the average of the endpoint's coordinates, mathematically:

mp=((x1+x2)/2, (y1+y2)/2)

In this case:

(-4,6)=((8+x)/2, (-2+y)/2)

(-8,12)=((8+x),(y-2))

(-16, 14)=(x,y)

Answer 2

Answer: (-16, 14)

Explanation:

We know that the coordinates of mid point of a line segment having end points (a,b) and (m,n) is given by :-

`x=(a+m)/(2)\ \ ,\ \ y=(b+n)/(2)`

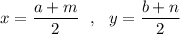
Given : The midpoint of MN is point P at (-4, 6). If point M is at (8, -2).

Let the coordinates of N be (a,b).

Then by using the above formula, we have

`-4=(8+a)/(2)\ \ ,\ \ 6=(-2+b)/(2)`

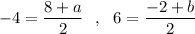
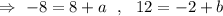`\Rightarrow\ -8=8+a\ \ ,\ \ 12=-2+b` [Multiplying 2 on both sides]

`\Rightarrow\ a=-8-8=-16\ \ ,\ \ b=12+2=14`

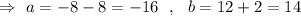
Hence, the coordinates of point N = (-16, 14)