Question

A projectile is launched from ground level with an initial velocity of Vo feet per second. Neglecting air resistance, its height in feet t seconds after launch is given bys = - 16t^2 + Vot. Find the time(s) that the projectile will (a) reach a height of 96ft and (b) return to the ground when Vo = 112 feet per second.(a) Find the time(s) that the projectile will reach a height of 96ft when vo = 112 feet per second. Select the correct choice below and, if necessary, fill in the answerto complete your choice.(A) ___ seconds (Use a comma to separate answers as needed.)(B) The projectile does not reach 96 feet.

Answer 1

To solve this question, follow the steps below.

Step 01: Substitute V0 by 112 feet per second.

`s=-16t^2+112t`

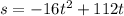
Step 02: Find t when s = 96.

To do it, first, substitute s by 96:

`96=-16t^2+112t`

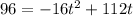
Now, subtract 96 from both sides of the equation:

`\begin{gathered} 96-96=-16t^2+112t-96 \\ 0=-16t^2+112t-96 \\ -16t^2+112t-96=0 \end{gathered}`

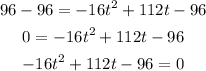
Use the Quadratic formula to find t.

For a equation ax² + bx + c = 0, x is:

`x=(-b\pm√(b^2-4ac))/(2a)`

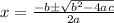
In this question,

a = -16

b = 112

c = -96

Substituting in the equation to find t:

Time to reach 96 feet = 1 or 6 seconds.

Step 03: Find the time when the projectile will return to the ground.

When the projectile return to the ground, s = 0.

Then, substitute s by 0 and find x using the same equation from step 02.

`\begin{gathered} 0=-16t^2+112t \\ -16t^2+112t=0 \end{gathered}`

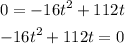
Then,

a = -16

b = 112

c = 0

Substituting in the quadratic formula:

`\begin{gathered} x=(-b\pm√(b^2-4ac))/(2a) \\ t=(-112\pm√(112^2-4*(-16)*0))/(2*(-16)) \\ t=(-112\pm√(12544-0))/(-32) \\ t=(-112\pm√(12544))/(-32) \\ t=(-112\pm112)/(-32) \\ t_1=(-112-112)/(-32)=(-224)/(-32)=7 \\ t_2=(-112+112)/(-32)=0 \end{gathered}`

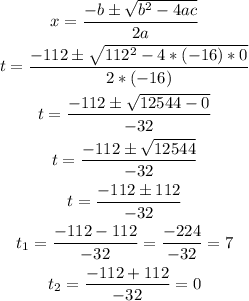
The projectile will reach the ground when t = 0 and when t = 7. t = 0 is the time when the projectile is launched and t = 7 is when the projectile returns to the ground.

(b) So, the projectile returns to the ground when t = 7 seconds.

Answer:

(a) The projectile reached 96 feet when t = 1 or t = 6 seconds.

(b) The projectile returns to the ground when t = 7 seconds.