Question

The triangles above are similar. Write a similarity statement and solve for x if possible

Answer 1

the have three sides and they also have three corners those are the similarites of both triangles

Answer 2

Similar triangles means that they have proportional sides and congruent angles.

According to the similarity, we can form the following proportion between corresponing sides

`(7x)/(5x+2)=(XY)/(21)`

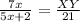
However, we need to find an expression for XY using Pythagorean's Theorem:

`(7x)^(2) =28^(2) +XY^(2)\\XY^(2)=49x^(2) -784\\XY=\sqrt{49(x^(2)-16)} =7\sqrt{x^(2) -16}`

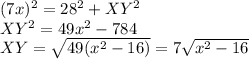
Replacing it in the proportion, we have

`(7x)/(5x+2)=\frac{7\sqrt{x^(2)-16 } }{21}\\21x=(5x+2)\sqrt{x^(2)-16 }\\(21x)^(2) =(5x+2)^(2)(\sqrt{x^(2)-16 })^(2) \\441x^(2) =(5x+2)^(2)(x^(2) -16)\\441x^(2) =25x^4+20x^3-396x^2-320x-64\\25x^4+20x^3-837x^2-320x-64=0`

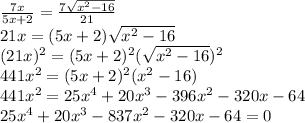
Using a calculator, we have

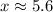`x \approx 5.6` and
`x\approx -6.01`.

Therefore, the solution is
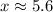`x \approx 5.6`.