Question

perform the symmetry test on each of the following hyperbola (b) 9x2 - y2 = 9 (d) 25x2 - 36y2 = 900 (f) -25x2 + 4y2 = 100 (h) -x2 + 4y2 = 16 tho

Answer 1

Given 9 x^2 - y^2 = 9

Divide both sides by 9, we have:

`\begin{gathered} (x^2)/(1)\text{ - }(y^2)/(9)\text{ = 1} \\ (\text{ a,b ) = (1, 9) , radius = 1} \\ \end{gathered}`

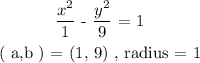
Axes of symmetry

There are two lines about which a hyperbola is symmetrical.

For the standard hyperbola y=1x, we see that if we replace x⇒y and y⇒x, we get y=1x. Similarly, if we replace x⇒−y and y⇒−x, the function remains the same.

Therefore the function is symmetrical about the lines y=x and y=−x.

The lines of symmetry are ; x = 0, y=0.