Question

an arithmetic sequence has a1 = 5 and a5 = 13. how many terms of this sequence must be added to obtain 60?

Answer 1

hmmm an arithmetic sequence is done by adding a value to the current term's value to get the next value, the added value is called the "common difference(d)"

so, let's do that to see what it might be then


`\bf \begin{array}{llll} term&value\\ \text{\textemdash\textemdash\textemdash}&\text{\textemdash\textemdash\textemdash}\\ a_1&5\\ a_2&5+d\\ a_3&5+d+d\\ & 5+2d\\ a_4&5+2d+d\\ &5+3d\\ a_5&5+3d+d\\ &5+4d \end{array} \\\\\\ \textit{we know }a_5=13\qquad thus\implies 5+4d=13\implies 4d=8 \\\\\\ d=\cfrac{8}{4}\implies \boxed{d=2}`

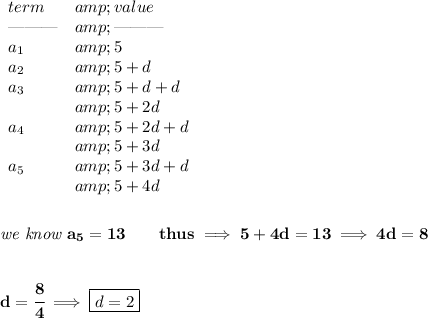
hmm I was looking how to go about using the Finite Sum equation... I don't see it happening with it though... so... we'd have to add them manually one by one till we get a sum of 60

let's get the term's values then, using d = 2

5 , 7, 9 , 11, 13, 15, 17, 19, 21
^

Answer 2

Answer with Step-by-step explanation:

An arithmetic sequence has a1 = 5 and a5 = 13.

the nth term of the arithmetic sequence is given by:

an= a1+(n-1)d where d is the common difference

a5= 5+ 4d

13= 5+4d

4d= 13-5

4d= 8

Dividing both sides by 4, we get

d=2

Also, sum of first n terms of an arithmetic progression is given by

s=
`(n)/(2)* (2a1+(n-1)d)`

60=
`(n)/(2)* (2* 5+2(n-1))`

120= n(10+2(n-1))

120= n(10+2n-2)

n(8+2n)=120

Dividing both sides by 2, we get

n(4+n)= 60

n²+4n-60=0

n²+10n-6n-60=0

n(n+10)-6(n+10)=0

(n-6)(n+10)=0

either n-6=0 or n+10=0

either n=6 or n= -10

n= -10 is not possible

So, n=6

Hence, number of terms that must be added to obtain 60 is:

6