Answer:
A. -a² + ab + 8a
B. -36 - 12a - a²
C. a² - b² - 4b - 4
Step-by-step explanation:
To multiply each expression, we need to apply the distributive property. So, for each expression, we get
A.
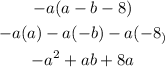
B.
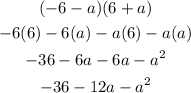
C.
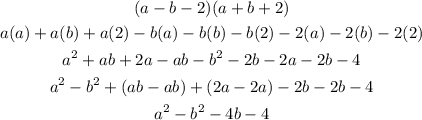
Therefore, the answers are
A. -a² + ab + 8a
B. -36 - 12a - a²
C. a² - b² - 4b - 4