Question

A cylinder has a height of 16 cm and a radius of 5 cm. A cone has a height of 12 cm and a radius of 4 cm. If the cone is placed inside the cylinder as shown, what is the volume of the air space surrounding the cone inside the cylinder? (Use 3.14 as an approximation of .)

Answer 1

Volume of Cylinder
= πr²h
= (3.14)(5)²(16)
= 1256 cm³

Volume of Cone
= 1/3πr²h
= 1/3(3.14)(4)²(12)
= 200.96 cm³

Volume of air space
= Volume of Cylinder - Volume of Cone
= 1256 - 200.96
= 1055.04 cm³
≈ 1055 cm³ (nearest whole number)

Answer 2

Explanation:

Alright, lets get started.

Lets find the volume of cylinder :
`\pi r^2h`

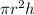
volume of cylinder =
`\pi (5)^2*16=400\pi`

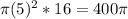
Lets find the volume of cone :
`(1)/(3)\pi r^2h`

volume of cone =
`(1)/(3)\pi 4^2*12=64\pi`

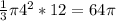
Hence the volume of air space will be volume of cylinder subtracted by volume of cone.

Hence the volume of air space =
`400\pi -64\pi`

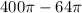
Hence the volume of air space =
`336\pi`

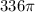
Plugging the value of π as 3.14

volume of air space =
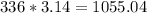`336*3.14=1055.04` : Answer

Hope it will help :)