Question

A rectangle with an area of x2 – 4x – 12 square units is represented by the model. What side lengths should be used to model the rectangle? (x + 2) and (x – 6) (x + 6) and (x – 2) (x + 2) and (x – 10) (x + 10) and (x – 2)

Answer 1

As long as that first number in the equation is x squared then the answer is
(x+2) and (x-6)

Answer 2

Answer: The correct option is (A)
`(x+2)~~\textup{and}~~(x-6).`

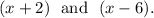
Step-by-step explanation: The area of a rectangle is given as follows:

`A=x^2-4x-12~\textup{square units.}`

We are to find the side lengths that should be used to model the rectangle.

The AREA of a rectangle is the PRODUCT of its length and width.

So, in order to find the dimensions, we need to factorize the expression for the area.

The factorization is as follows:

`A\\\\=x^2-4x-12\\\\=x^2-6x+2x-12\\\\=x(x-6)+2(x-6)\\\\=(x+2)(x-6).`

Therefore, the dimensions of the rectangle are
`(x+2)~~\textup{and}~~(x-6).`

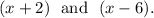
Option (A) is correct.