Question

I have to take a pic of the question

Answer 1

`y=(2)/(3)x+(4)/(3)`

Step-by-step explanation

We want to find the equation of the straight line given on the graph.

The general form of a linear equation is given as:

`y=mx+b`

where m = slope; b = y-intercept

To find the slope, we apply the formula for slope:

`m=(y_2-y_1)/(x_2-x_1)`

where (x1, y1) and (x2, y2) are two points on the line

Let us pick (1,2) and (4,4)

Therefore, the slope is:

`m=(4-2)/(4-1)=(2)/(3)`

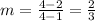
To find the equation, we now apply the point-slope formula:

`y-y_1=m(x-x_1)`

Therefore, we have that the equation of the line is:

`\begin{gathered} y-2=(2)/(3)(x-1) \\ y-2=(2)/(3)x-(2)/(3) \\ y=(2)/(3)x-(2)/(3)+2 \\ y=(2)/(3)x+(4)/(3) \end{gathered}`

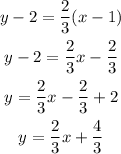
That is the equation of the line.