Question

What value of a, in degrees will refute Frank’s claim a= degrees?

Answer 1

Frank is comparing two triangles.

Triangle 1:

The triangle has 36 degrees and 51 degrees. We can find the third angle (k):

`\begin{gathered} 36+51+k=180\text{ (Sum of angles in a triangle)} \\ 87+k=180\text{ (Subtract 87 from both sides)} \\ k=180-87 \\ \therefore k=93 \end{gathered}`

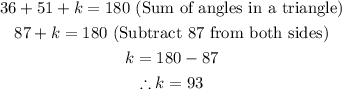
Therefore, the 3 angles of triangle 1 are: 36, 51 and 93

Triangle 2:

The second triangle has 36 degrees and angle a. Let us assume the third angle is also k:

`\begin{gathered} 36+a+k=180\text{ (Sum of angles in a triangle)} \\ a+k=180-36 \\ a+k=144 \end{gathered}`

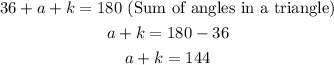
This means that the sum of the remaining unknown angles of triangle 2 is: 144.

Frank claims that since a is not 51, then the two triangles are not similar.

In order to have similar triangles, at least 2 angles must be equal.

3