Question

Consider the quadrilateral deZ23W112°Х23YWhat is the measure of angle Z?

Answer 1

Ok, in the pic we can see that tha quadrilateral is an isosceles trapezoid, so we know that:

The angles of the bases must have the same measure two by two and the angles adjacent to the opposite bases are supplementary. So, ising this, we get:

The measure of W= measure of X=112° and this mean also that angles Y and Z are equal.

So we have:

`W+X+Y+Z=360\degree`
`112\degree+112\degree+2Y=360\degree`
`224\degree+2Y=360\degree`

`2Y=360\degree-224\degree`
`2Y=136\degree`
`Y=(136\degree)/(2)=68\degree`

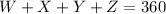
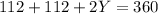
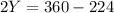
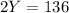
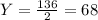
The measure of angles Y and Z are 68° each one of them.