We'll solve question 1.
a)
Simplifying,
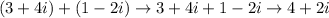
b)
Remember that we can express any complex number
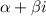
on the plane as

Thereby, our imaginary number 4 + 2i on the plane would look as following:
c)
Since we already have rectangular coordinates for our complex number, we can rewrite it in polar coordinates. Remember that to put any set of rectangular coordinates (x,y) on the polar plane, we use the following:
![\begin{gathered} r=\sqrt[]{x^2+y^2} \\ \theta=\tan ^(-1)((y)/(x)) \end{gathered}](https://img.qammunity.org/qa-images/2023/formulas/mathematics/college/7liwijtft0kjrvctafzx.png)
This way,
![r=\sqrt[]{4^2+2^2}\rightarrow r=\sqrt[]{20}\Rightarrow r=2\text{ }\sqrt[]{5}](https://img.qammunity.org/qa-images/2023/formulas/mathematics/college/715vbqat0bpw7gt3mlkh.png)
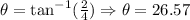
Therefore, our complex number in polar coordinates would be
![2\text{ }\sqrt[]{5}\text{ }\angle26.57](https://img.qammunity.org/qa-images/2023/formulas/mathematics/college/ukb32ypx1atu5ci1aos4.png)
d)
The plot would look as following: