Question

Graph f(θ) = sinθ where -2π ≤ θ ≤ 2π.

Answer 1

Given :

the function is,

`\: f\mleft(\theta\mright)\: =\: sin\theta\:`

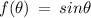
in the range,

`-2\pi\le\: \theta\: \le\: 2\pi`

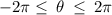
to find :

graph the function.

Step-by-step explanation:

a normal sin graph will look like the graph below,

here the graph, the graph has the interval,

`-2\pi\le\theta\le\: \: 2\pi\: \:`

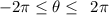
thus, the end point of the graph will be,

`\begin{gathered} (-2\pi,0) \\ \text{and } \\ (2\pi,0) \end{gathered}`

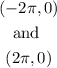
Final answer:

the graph will is as follows,