Question

A bench is located where the shadows casted by a 16 foot tall flagpole and 40-foot tall tree meet. If the bench is 18 feet from the flag pole, find the distance from the flagpole to the tree

Answer 1

The distance from the flagpole to the tree is 27 ft

Explanation:

Similar Triangles

Since the elevation angle from which the trees cast their shadows has the same measure and given the tree and the flagpole and the ground form right angles, both triangles are similar.

Similar triangles have their corresponding side lengths proportional. The ratio between the distances from the bench to the tree and flagpole and their heights is constant, i.e.:

`\displaystyle (16)/(18)=(40)/(x)`

Where x is the distance from the bench to the tree.

Solving for x:

`\displaystyle x=40\cdot (18)/(16)`

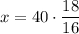
x = 45 feet

Thus the distance from the flagpole to the tree is 45 - 18 = 27 feet