Question

Given that the width of the cereal box is 8 centimeters, which expression could be the dimensions of the length and the height of the box shown?A (x+3)(2x+1)B (2x+3)(x+1)C (16x+8)(x+3)D (8x+24)(2x+1)

Answer 1

The given expression is

`16x^2+56x+24=0`

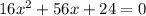
To determine the dimensions of the length and the height, we have to factor the expression.

We know that a = 16, b = 56, and c = 24. Remember that a is the coefficient of the quadratic variable, b is the coefficient of the linear variable, and c is the independent term.

Now, we use the quadratic formula

`x_(1,2)=\frac{-b\pm\sqrt[]{b^2-4ac}}{2a}`

Let's replace all the values

`\begin{gathered} x_(1,2)=\frac{-56\pm\sqrt[]{56^2-4\cdot16\cdot24}}{2\cdot16} \\ x_(1,2)=\frac{-56\pm\sqrt[]{3136-1536}}{32}=\frac{-56\pm\sqrt[]{1600}}{32} \\ x_(1,2)=(-56\pm40)/(32) \end{gathered}`

We have two solutions here

`\begin{gathered} x_1=(-56+40)/(32)=(-16)/(32)=-(1)/(2) \\ x_2=(-56-40)/(32)=(-96)/(32)=-3 \end{gathered}`

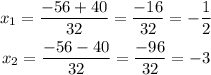
If we express them as factors, it would be

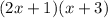
`(2x+1)(x+3)`Which is equivalent to A.