2 Answers

Jeffm · Answer 1 · 2018-12-21T22:39:35+0000

Answer:

The ratio of their surface area is given by:

9:64

We know that if two solids are similar such that the ratio of their sides or edges is given by:
(a)/(b)

Then the ratio of the surface area of the two solids is given by:

(a^2)/(b^2)

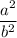
Here we have:

(a)/(b)=(3)/(8)

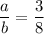
Hence, on squaring both side of the equality we obtain:

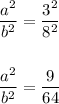
$(a^2)/(b^2)=(3^2)/(8^2)\\\\\\(a^2)/(b^2)=(9)/(64)$

Hence, the ratio of the surface area of two similar solids is:

9:64