Question

To the nearest hundredth, what is the length of 

line segment AB ?

Drag your answer into the box.
The length of line segment AB is approximately units.
A.9.54 B.10.44 C.13.00 D.13.15
A graph with point A at (1, -1) and point B at (-9, -4)

Answer 1

Answer: The correct option is (B) 10.44.

Step-by-step explanation: We are given a graph that has a point A at (1, -1) and a point B at (-9, -4).

We are to find the length of the line segment AB to the nearest hundredth.

The length of the line segment AB is given by the distance between the end-points A and B.

DISTANCE FORMULA: The distance between two points with co-ordinates (a, b) and (c, d) is given by

`D=√((c-a)^2+(d-b)^2).`

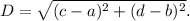
Therefore, the distance between the points A(1, -1) and B(-9, -4) is

`AB\\\\=√((-9-1)^2+(-4-(-1))^2)\\\\=√(100+9)\\\\=√(109)\\\\=10.44~\textup{units}.`

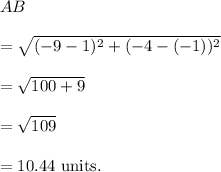
Thus, the length of the line segment AB is 10.44 units.

Option (B) is CORRECT.