Question

Problem 1.Suppose a quadratic function f(x) has its vertex at x = 0.5. Below is a table of values for f(x):a. What are the zeros of f(x)?= 0 and x=0b. What is f(-4)?f(-4)=c. Find the y-coordinate of the vertex of f(x).f(0.5) -xf(x)-2 1 24 -2 0

Answer 1

The distance from the zeros of a function to its vertex is the same; use the given zero (at x=2) and the x-value of the vertex (x=0.5) to find the other zero:

`\begin{gathered} distance:2-0.5=1.5 \\ zeros\text{ }are\text{ }at:0.5\pm1.5 \\ \\ 0.5-1.5=-1 \\ 0.5+1.5=2 \end{gathered}`

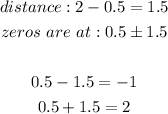
a, The zeros are:

x= -1 and x=2

b. Find the equation of f(x) using the zeros:

`\begin{gathered} x=-1 \\ x+1=0 \\ \\ x=2 \\ x-2=0 \\ \\ f(x)=a(x+1)(x-2) \end{gathered}`

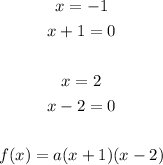
Use one of the points in the table to find a:

`\begin{gathered} (-2,4) \\ \\ 4=a(-2+1)(-2-2) \\ 4=a(-1)(-4) \\ 4=4a \\ 4/4=a \\ \\ a=1 \end{gathered}`

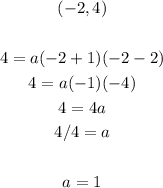
Function is:

`f(x)=(x+1)(x-2)`

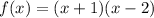
Use the function to find f(-4):

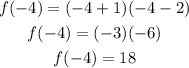
`\begin{gathered} f(-4)=(-4+1)(-4-2) \\ f(-4)=(-3)(-6) \\ f(-4)=18 \end{gathered}`f(-4)=18

c, Use the equation of the function to find f(0.5)

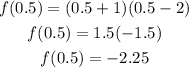
`\begin{gathered} f(0.5)=(0.5+1)(0.5-2) \\ f(0.5)=1.5(-1.5) \\ f(0.5)=-2.25 \end{gathered}`f(0.5)=-2.25