Question

What is the equation of the line containing the points (5,2), (10,4), and (15, 6)? A. y= 2/5x B. y = x - 3 C. y= 1/5x+1

Answer 1

Given the points (5,2) and (10,4), we can find the slope of the line that passes through them with the following formula:

`m=(y_2-y_1)/(x_2-x_1)`

in this case we have the following:

`\begin{gathered} (x_1,y_1)=(5,2) \\ (x_2,y_2)=(10,4) \\ \Rightarrow m=(4-2)/(10-5)=(2)/(5) \\ m=(2)/(5) \end{gathered}`

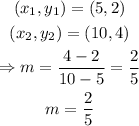
now that we have that the slope is m = 2/5, we can find the equation of the line using the first point and the point-slope formula:

`\begin{gathered} y-y_1=m(x-x_1) \\ \Rightarrow y-2=(2)/(5)(x-5)=(2)/(5)x-5\cdot((2)/(5))=(2)/(5)x-2 \\ \Rightarrow y=(2)/(5)x-2+2=(2)/(5)x \\ y=(2)/(5)x \end{gathered}`

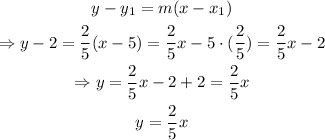
therefore, the equation of the line that contains the points (5,2) and (10,4) is y = 2/5 x