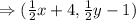Question

Describe the similarity transformation that maps the preimage to the image. I need help with question 4

Answer 1

The coordinates of the vertices of the original shape are given to be:

`\begin{gathered} L\Rightarrow(6,6) \\ M\Rightarrow(0,4) \\ N\Rightarrow(0,6) \end{gathered}`

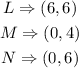
The transformed shape coordinates are given to be:

`\begin{gathered} L^(\prime)\Rightarrow(7,2) \\ M^(\prime)\Rightarrow(4,1) \\ N^(\prime)\Rightarrow(4,2) \end{gathered}`

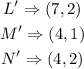
On observation, the shapes show a dilation and a shift in position.

The image has a scale factor of 1/2, meaning it is reduced by 1/2. Therefore, the original coordinates will be reduced to give:

`\begin{gathered} L^(\doubleprime)\Rightarrow(1)/(2)(6,6)=(3,3) \\ M^(\doubleprime)\Rightarrow(1)/(2)(0,4)=(0,2) \\ N^(\doubleprime)\Rightarrow(0,6)\Rightarrow(0,3) \end{gathered}`

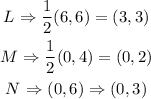
The translation of the initial image can be gotten by subtracting the corresponding coordinates:

`\begin{gathered} L^(\prime)-L^(\doubleprime)=(7-3,2-3)=4,-1 \\ M^(\prime)-M^(\doubleprime)=(4-0,1-2)=4,-1 \\ N^(\prime)-N^(\doubleprime)=(4-0,2-3)=4,-1 \end{gathered}`

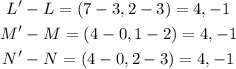
All the differences are the same. This means that the image moves to the right by 4 units and down by 1 unit.

The dilation rule with a scale factor of k is given to be:

`(x,y)\to(kx,ky)`

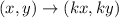
The translation rule for a units to the right and b units down is given to be:

`(x,y)\to(x+a,y-b)`

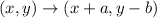
Combining both rules, we have:

`(x,y)\to(kx+a,ky-b)`

Given:

`\begin{gathered} k=(1)/(2) \\ a=4 \\ b=1 \end{gathered}`

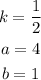
Therefore, the transformation is given to be:

`\Rightarrow((1)/(2)x+4,(1)/(2)y-1)`