Question

Find the exact values of the six trigonometric functions functions of the angles 0 shown in the figure. Sin, cos, tan, csc, sec and cot (Use the pythagorean theorem to find the third side of the triangle)

Answer 1

Step-by-step explanation:

Let x represent the length of the third side of the given triangle.

We can go ahead and determine the value of x using the Pythagorean theorem as seen below;

`\begin{gathered} 41^2=x^2+40^2 \\ 1681=x^2+1600 \\ x^2=1681-1600 \\ x^2=81 \\ x=\sqrt[]{81} \\ x=9 \end{gathered}`

So the length of the third side of the triangle is 9

We can now determine the value of sine theta as seen below;

`\begin{gathered} \sin \theta=\frac{opposite\text{ side to angle }\theta\text{ }}{\text{hypotenuse}}=(40)/(41) \\ \therefore\sin \theta=(40)/(41) \end{gathered}`

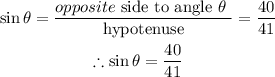
We can see that sine theta is 40/41

Let's determine the value of cosine theta as seen below;

`\begin{gathered} \cos \theta=\frac{\text{adjacent side to angle }\theta}{\text{hypotenuse}}=(9)/(41) \\ \therefore\cos \theta=(9)/(41) \end{gathered}`

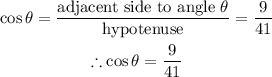
So cosine theta is 9/41

Let's determine the value of tangent theta as seen below;

`\begin{gathered} \tan \theta=\frac{opposite\text{ side to angle }\theta}{\text{adjacent side to angle }\theta}=(40)/(9) \\ \tan \theta=(40)/(9) \end{gathered}`

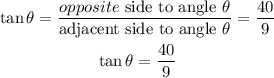
So tangent theta is 40/9

Let's now determine the value of cosecant theta as seen below;

`\begin{gathered} \csc \theta=(1)/(\sin\theta)=(1)/((40)/(41))=1/(40)/(41)=1*(41)/(40)=(41)/(40) \\ \therefore\csc \theta=(41)/(40) \end{gathered}`

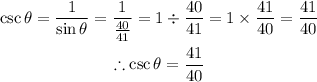
So the value of cosecant theta is 41/40

Let's determine the value of secant theta as seen below;

`\begin{gathered} \sec \theta=(1)/(\cos\theta)=(1)/((9)/(41))=1/(9)/(41)=1*(41)/(9)=(41)/(9) \\ \therefore\sec \theta=(41)/(9) \end{gathered}`

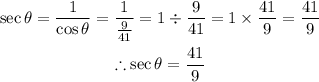
So the value of secant theta is 41/9

Let's determine the value of cotangent theta as seen below;

`\begin{gathered} \cot x=(1)/(\tan x)=(1)/((40)/(9))=1/(40)/(9)=1*(9)/(40)=(9)/(40) \\ \cot x=(9)/(40) \end{gathered}`

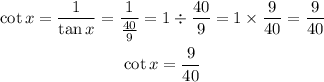
So the value of cotangent theta is 9/40