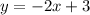Question

This is grade 10 math. Chapter 2: Analytic Geometry - Line Segments and CirclesDescribe all points that are the same distance from points A(-3,-1) and B(5,3)It’s question 3 from attached screenshot.The answer at the back of text book is y=-2x+3Thank you!

Answer 1

Given:

The given points are A(-3,-1) and B(5,3).

Required:

We need to find the set of all points that are the same distance from the points A(-3,-1) and B(5,3).

Step-by-step explanation:

Recall that the set of points that are the same distance from the point A(-3,-1) and (5,3) is the perpendicular bisector of the line segment AB.

Consider the slope formula.

`slope=(y_2-y_1)/(x_2-x_1)`
`Substitute\text{ }y_2=3,y_1=-1,x_2=5,\text{ and }x_1=-3\text{ in the formula to find the slope of AB.}`

`slope=(3-(-1))/(5-(-3))=(3+1)/(5+3)=(4)/(8)=(1)/(2)`

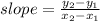
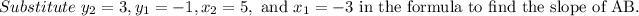
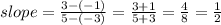
We get the slope of the line segment AB is 1/2.

The slope of the perpendicular is the negative reciprocal of the slope of the line segment AB.

`The\text{ negative reciprocal of }(1)/(2)=-2.`

`The\text{ slope of the perpendicular line is -2.}`

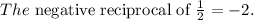
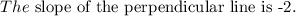
The perpendicular line is passing through the midpoint of A(-3,-1) and B(5,3).

Consider the midpoint formula.

`midpoint=((x_1+x_2)/(2),(y_1+y_2)/(2))`

`Substitute\text{ }y_2=3,y_1=-1,x_2=5,\text{ and }x_1=-3\text{ in the formula to find the midpoint of AB.}`

`midpoint=((-3+5)/(2),(-1+3)/(2))`
`midpoint=((2)/(2),(2)/(2))`
`midpoint=(1,1)`

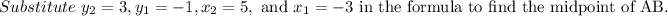
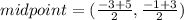
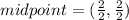
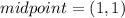
Consider the general form of the line equation.

`y=mx+b`

Substitute m=-2, x=1, and y=1 in the line equation to find b.

`1=(-2)(1)+b`
`1=-2+b`

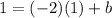
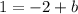
Add 2 to both sides of the equation.

`1+2=-2+b+2`
`3=b`

Substitute m=-2 and b=3 in the line equation.

`y=-2x+3`

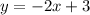
We get the perbenticular bisector y =-2x+3.

Final answer:

`y=-2x+3`