Question

Compare Rates (Linear Representations)

Answer 1

Question:

Solution:

Remember that the equation of a line is given by:

`y\text{ = mx+b}`

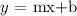
where m is the slope of the line. Now, the given line is:

`y\text{ = -3x+3}`

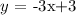
thus, the slope of this line is m= -3. Now, the problem asks us to find which of the given relationships represents a function with a lesser slope than the given line. To do this, remember that the slope of a line is given by the following equation:

`m\text{ = }(Y2-Y1)/(X2-X1)`

where (X1, Y1) and (X2,Y2) are points on the line or data in a file of the table that represent a line.

therefore, we are going to calculate the slope for each relationship given in the problem:

For A:

(X1,Y1) = (0,-1)

(X2,Y2)= (-1,5)

then the slope is:

`m\text{ = }(Y2-Y1)/(X2-X1)\text{ = }(5-(-1))/(-1-0)\text{ =}(5+1)/(-1)\text{ = -6}`

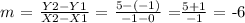
note that

`-6<-3`

For B:

(X1,Y1) = (-6,-3)

(X2,Y2)= (-4,-1)

then the slope is:

`m\text{ = }(Y2-Y1)/(X2-X1)=\text{ }(-1-(-3))/(-4-(-6))=\text{ }(-1+3)/(-4+6)\text{ = }(2)/(2)\text{ = 1}`

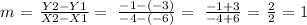
note that:

`1>-3`

For C:

(X1,Y1) = (-1,2)

(X2,Y2)= (-2,3)

then the slope is:

`m\text{ = }(Y2-Y1)/(X2-X1)\text{ = }(3-2)/(-2-(-1))\text{ = }(1)/(-2+1)=\text{ -}(1)/(1)=-1`

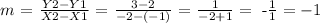
note that:

`-1>-3`

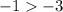
Finally:

For D:

(X1,Y1) = (-4,7)

(X2,Y2)= (-2,1)

then the slope is:

`m\text{ = }(Y2-Y1)/(X2-X1)=(1-7)/(-2-(-4))=\text{ }(-6)/(-2+4)=(-6)/(2)\text{ = -3}`

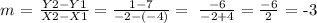
note that:

`-3\text{ = -3}`

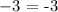
so that, we can conclude that the correct answer is A:

that is because the slope of graph A is lesser than the given line:

`-6<-3`