Question

How does the graph of y = sec(x + 3) – 7 compare with the graph of y = sec(x)?

Answer 1

C

Explanation:

Answer 2

The function y = sec(x) shifted 3 units left and 7 units down .

Explanation:

Given the function: y = sec(x)

• If k is any positive real number, then the graph of f(x) - k is the graph of y = f(x) shifted downward k units.

• If p is a positive real number, then the graph of f(x+p) is the graph of y=f(x) shifted to the left p units.

The function
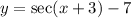`y = \sec(x+3)-7` comes from the base function y= sec(x).

Since 3 is added added on the inside, this is a horizontal shift Left 3 unit, and since 7 is subtracted on the outside, this is a vertical shift down 7 units.

Therefore, the transformation on the given function is shifted 3 units left and 7 units down