Step 1
Given;
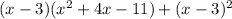
Required; To factorize the problem
Step 2
Find the GCF
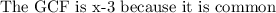
Hence, we will factorize thus and have;
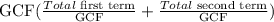
Applying this we will have;
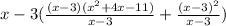
![\begin{gathered} (x-3)((x^2+4x-11)_{}+(x-3)) \\ (x-3)(x^2+4x+x-11-3)--\text{ open bracket and add like terms} \\ (x-3)(x^2+5x-14) \end{gathered}]()
Therefore, we will now factorize (x²+5x-14) by finding the terms that when added together gives 5x and when multiplied together gives -14x²

Replace 5x with (7x-2x)
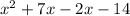
Grouping the four terms in twos, that is the first two as a pair and the remaining two as the other pair, we can bring out common terms from each pair. This is shown below:
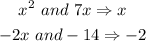
Therefore, using the factoring method shown in Step 2, we have the expression to be:

Collecting the like terms, we, therefore, have the factorized expression to be:
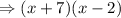
Therefore the full factorized expression will be;
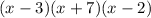
The answer will therefore be ; (x-3)(x+7)(x-2)