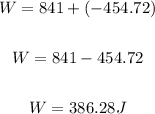Question

A farmer is using a rope and pulley to lift a bucket of water from the bottom of a well that is hy = 14.5 m deep. The farmer uses a force F1 = 58 N to pull the bucket of water directly upwards. The total mass of the bucket of water is mb + mw = 3.2 kg.Help with b, c, and d

Answer 1

`\begin{gathered} (b)\text{ }841J \\ \\ (c)\text{ }-454.72J \\ \\ (d)\text{ }386.28J \end{gathered}`

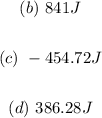
Step-by-step explanation

Parameters given:

Depth of the well, hy = 14.5 m

Force of pull, F1 = 58 N

The total mass of the bucket of water, mb + mw = 3.2 kg

(b) The amount of work done by the farmer on the bucket, we have to apply the formula for work done i.e. the product of force applied and distance traveled:

`W_f=F_1*h_y`

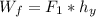
Therefore, the work done by the farmer is:

`\begin{gathered} W_f=58*14.5 \\ \\ W_f=841J \end{gathered}`

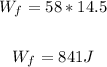
(c) To find the work done by gravity, apply the formula:

`W_g=-(m_b+m_w)gh_y`

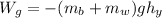
where g = acceleration due to gravity

Therefore, the work done by gravity is:

`\begin{gathered} W_g=-3.2*9.8*14.5 \\ \\ W_g=-454.72J \end{gathered}`

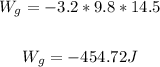
(d) The total work done by the two forces is the sum of the work done by the farmer and the work done by gravity:

`W=W_f+W_g`

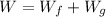
Therefore, the total work done is:

`\begin{gathered} W=841+(-454.72) \\ \\ W=841-454.72 \\ \\ W=386.28J \end{gathered}`