Question

Solve for the remaining angles and side of the two triangles that can be created. Round to the nearest hundredth:C = 35", a = 8.c = 5

Answer 1

Step-by-step explanation

The sum of the three internal angles of a triangle is equal to 180°. Then for a triangle with angles A, B and C we get:

`A+B+C=180^(\circ)`

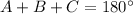
Considering the sides of the triangle are a, b and c and they are opposite to angles A, B and C respectively the law of sines states that:

`(a)/(\sin A)=(b)/(\sin B)=(c)/(\sin C)`

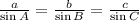
Now let's use these properties to find the other two possible triangles. From the law of sines we get:

`(8)/(\sin A)=(b)/(\sin B)=(5)/(\sin35^(\circ))`

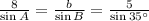
We can build an equation for A:

`(8)/(\sin A)=(5)/(\sin35^(\circ))`

We can invert both sides of this equation:

`(\sin A)/(8)=(\sin 35^(\circ))/(5)`

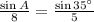
And we multiply both sides by 8:

`\begin{gathered} (\sin A)/(8)\cdot8=(\sin35^(\circ))/(5)\cdot8 \\ \sin A=(8)/(5)\sin 35^(\circ) \end{gathered}`

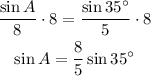
We can use the arcsin function to find A:

`\begin{gathered} \sin ^(-1)(\sin A)=\sin ^(-1)((8)/(5)\sin 35^(\circ)) \\ A=66.60^(\circ) \end{gathered}`

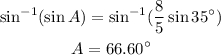
However that's not the only possible value of A. A can be acute (smaller than 90°) or obtuse (greater than 90°). This is the value of A if it's acute. In order to find the value of A when it's obtuse we have to find an angle with a sine that is equal to that of A.

It's important to remember that for any angle x in the first quadrant [0°,90°) there's another angle in the second quadrant [90°,180°) that has the same sine. This angle is given by:

`180^(\circ)-x`

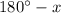
So the obtuse value of A is:

`180^(\circ)-66.60^(\circ)=113.40^(\circ)`

Now let's find B and b in each case.

First if A=66.60° the sum of A, B and C is:

`\begin{gathered} A+B+C=180^(\circ) \\ 66.60^(\circ)+B+35^(\circ)=180^(\circ) \\ B+101.60^(\circ)=180^(\circ) \end{gathered}`

Then we substract 101.6° from both sides:

`\begin{gathered} B+101.60^(\circ)-101.60^(\circ)=180^(\circ)-101.60^(\circ) \\ B=78.40^(\circ) \end{gathered}`

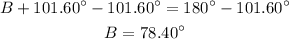
Then the law of sines looks like this:

`(8)/(\sin66.60^(\circ))=(b)/(\sin78.40^(\circ))=(5)/(\sin35^(\circ))`

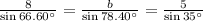
So we have the following equation for b:

`(b)/(\sin78.40^(\circ))=(5)/(\sin35^(\circ))`

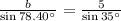
We multiply both sides by sin(78.40°) and we find b:

`\begin{gathered} (b)/(\sin78.40^(\circ))\cdot\sin 78.40^(\circ)=(5)/(\sin35^(\circ))\sin 78.40^(\circ) \\ b=(5)/(\sin35^(\circ))\sin 78.40^(\circ)=8.54 \end{gathered}`

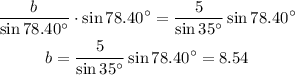
Now let's do the same for the obtuse value of A: 113.40°. The sum of the internal angles is:

`\begin{gathered} A+B+C=180^(\circ) \\ 113.40^(\circ)+B+35^(\circ)=180^(\circ) \\ B+148.40^(\circ)=180^(\circ) \end{gathered}`

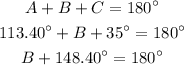
We substract 148.40° from both sides:

`\begin{gathered} B+148.40^(\circ)-148.40^(\circ)=180^(\circ)-148.40^(\circ) \\ B=31.6^(\circ) \end{gathered}`

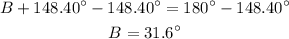
Then the law of sines is:

`(8)/(\sin113.40^(\circ))=(b)/(\sin31.60^(\circ))=(5)/(\sin35^(\circ))`

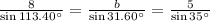
Then we get this equation for b:

`(b)/(\sin31.60^(\circ))=(5)/(\sin35^(\circ))`

And we multiply both sides by 31.60°:

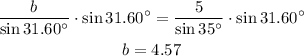
`\begin{gathered} (b)/(\sin31.60^(\circ))\cdot\sin 31.60^(\circ)=(5)/(\sin35^(\circ))\cdot\sin 31.60^(\circ) \\ b=4.57 \end{gathered}`Answers

The answers for triangle 1 are:

A = 66.60°

B = 78.40°

b = 8.54

The answers for triangle 2 are:

A = 113.40°

B = 31.60°

b = 4.57