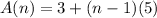Solution:
Given:
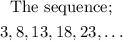
The sequence given is an arithmetic progression because it increases by a common difference.
Hence, the function rule for the sequence will follow that of an arithmetic progression (A.P).
The nth term of an A.P is given by;

For the sequence given;

Therefore, the function rule for the sequence is;