Question

A Physics student is thrown horizontally at a velocity of 12 m/s from the top of a cliff 68m high. How far from the base of the cliff does the student land? (2 sig figs)

Answer 1

`45m`

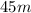
Step-by-step explanation

To find how far the student lands, we first have to find the time of flight using the equation that governs vertical motion:

`y-y_0=v_(0y)t+(1)/(2)a_yt^2`

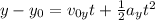
where y0 = initial height; y = final height; voy = initial velocity in the y direction; t = time; ay = -g; g = acceleration due to gravity

From the question, we have that:

`\begin{gathered} y=0 \\ y_0=68m \\ v_(0y)=0m/s^{} \\ t=\text{?} \\ g=9.8m/s^2 \end{gathered}`

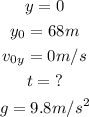
Therefore, we have that:

`\begin{gathered} 0-y_0=0-(1)/(2)gt^2 \\ \Rightarrow t^2=(2y_0)/(g) \\ t=\sqrt[]{(2y_0)/(g)} \\ t=\sqrt[]{(2\cdot68)/(9.8)} \\ t=3.73\text{ seconds} \end{gathered}`

Now, we can apply the equation that governs horizontal motion to find the distance from the base of the cliff:

`x-x_0=v_(0x)t+(1)/(2)a_xt^2_{}_{}_{}_{}`

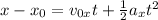
where x = distance from bottom of cliff; x0 = starting position; v0x = initial velocity in the x-direction; ax = acceleration in the x-direction

From the question, we have that:

`\begin{gathered} x_0=0m \\ a_x=0m/s^2 \\ x=\text{?} \\ v_(0x)=12\text{ m/s} \end{gathered}`

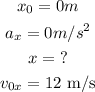
Therefore, the distance of the student from the base of the cliff is:

`\begin{gathered} x-0=v_(0x)t+0 \\ \Rightarrow x=v_(0x)t \\ x=12\cdot3.73 \\ x\approx45m \end{gathered}`

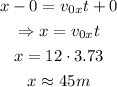
That is the answer (to 2 significant figures).