hello
to solve this question, we can simply use the theorem "angle on a straight line is equal to 180 degrees"
for the first polygon
to solve for x, y and z, we should simply subtract the adjacent interior angle from 180 degrees
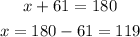
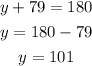

sum of exterior angles is
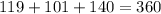
now we can proceed to the next polygon
now we can simply use the previous method for the last one
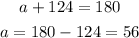

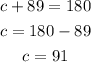
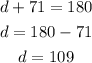
the sum of the exterior angles is

the next polygon is
then we proceed with the same method we've used before
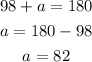
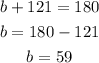
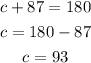
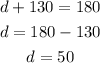

the sum of the exterior angles is

now we can solve for the last polygon
we can proceed to solve this through the previous method
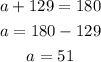
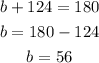
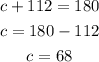

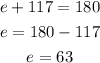
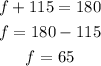
the sum of the exterior polygons is
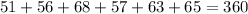
the sum of all the exterior angles of a regular polygon is equal to 360 degrees