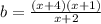Question

The area of a rectangle is given by the expression x2 + 5x + 4. If the length of one side is given by x + 2, what is the length of the other side?

Answer 1

If I'm understanding correctly, then...

(X^2+5x+4)/(x+2)=the length of the other side
Length= x+3+((-3)/(x+2))

Answer 2

The length of the other side is
`b=((x+4)(x+1))/(x+2)`

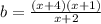
Explanation:

Given : The area of a rectangle is given by the expression
`x^2 + 5x + 4`. If the length of one side is given by x+2.

To find : What is the length of the other side?

Solution :

The formula of area of rectangle is

`A=length* breadth`

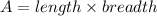
Where,
`A=x^2 + 5x + 4`

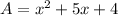
and one side length is
`l=x+2`

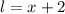
We have to fond other side i.e. breadth b

Substitute in the formula,

`x^2 + 5x + 4=x+2* b`

`b=(x^2 + 5x + 4)/(x+2)`

`b=(x^2+4x+x + 4)/(x+2)`

`b=((x+4)(x+1))/(x+2)`

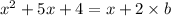
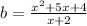
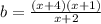
Therefore, The length of the other side is
`b=((x+4)(x+1))/(x+2)`