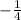Question

I need a short solution to this problem. (Calculus and Vectors homework)

Answer 1

Given the function f(x), the slope is evaluated by differentiating the f(x) function with respect to x.

Thus,

`\text{slope = }(df(x))/(dx)\text{ = f'(x)}`

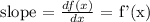
Given a function f(x) as

`f(x)\text{ = }\frac{4}{\sqrt[]{x+5}}`

Step 1:

Differentiate the f(x) function with respect to x.

`\begin{gathered} f(x)\text{ = }\frac{4}{\sqrt[]{x+5}}\text{ can also be written as} \\ f(x)\text{ = }4(x+5)^{-(1)/(2)} \end{gathered}`

differentiating the f(x) function, we have

`\begin{gathered} f(x)\text{ = }4(x+5)^{-(1)/(2)} \\ (df(x))/(dx)\text{ = -}(1)/(2)*4(x+5)^{-(1)/(2)-1} \\ \Rightarrow slope=f^(\prime)(x)=-2(x+5)^{-(3)/(2)} \end{gathered}`

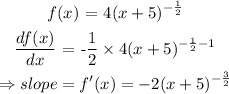
Step 2:

Evaluate the slope at the point (-1, 1).

The slope at the point (-1, 1) is evaluated by substituting the values of x and y into the f'(x) function.

Thus,

`\begin{gathered} x\text{ = -1, y = 1} \\ \text{substitute the values of x and y into f'(x)} \\ f^(\prime)(x)=-2(x+5)^{-(3)/(2)} \\ =-2(-1+5)^{-(3)/(2)} \\ =-2(4)^{-(3)/(2)} \\ =-2*(1)/(8)^{} \\ =-(1)/(4) \end{gathered}`

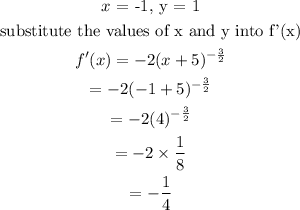
Hence, the slope of the function at the point (-1, 1) is

`-(1)/(4)`