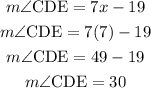Question

In ABCD, BD is extended through point D to point E, m BCD = (2x – 1)º,mZCDE = (7x – 19)°, and mZDBC = (x + 10)°. Find mZCDE.

Answer 1

Let's draw the figure:

CDE is the exterior angle to the Triangle BCD.

The two opposite interior to CDE is the angle B and angle C.

We know:

An exterior angle of a triangle is equal to the sum of the two opposite interior angles.

Thus, we can write

`\begin{gathered} 7x-19=x+10+2x-1 \\ \end{gathered}`

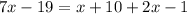
Now, let's solve for x:

`\begin{gathered} 7x-19=x+10+2x-1 \\ 7x-19=3x+9 \\ 7x-3x=9+19 \\ 4x=28 \\ x=(28)/(4) \\ x=7 \end{gathered}`

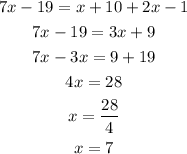
We want to find the angle CDE. Thus,

`\begin{gathered} m\angle\text{CDE}=7x-19 \\ m\angle\text{CDE}=7(7)-19 \\ m\angle\text{CDE}=49-19 \\ m\angle\text{CDE}=30\degree \end{gathered}`